Introduced to this in AP Calculus AB
What is it?
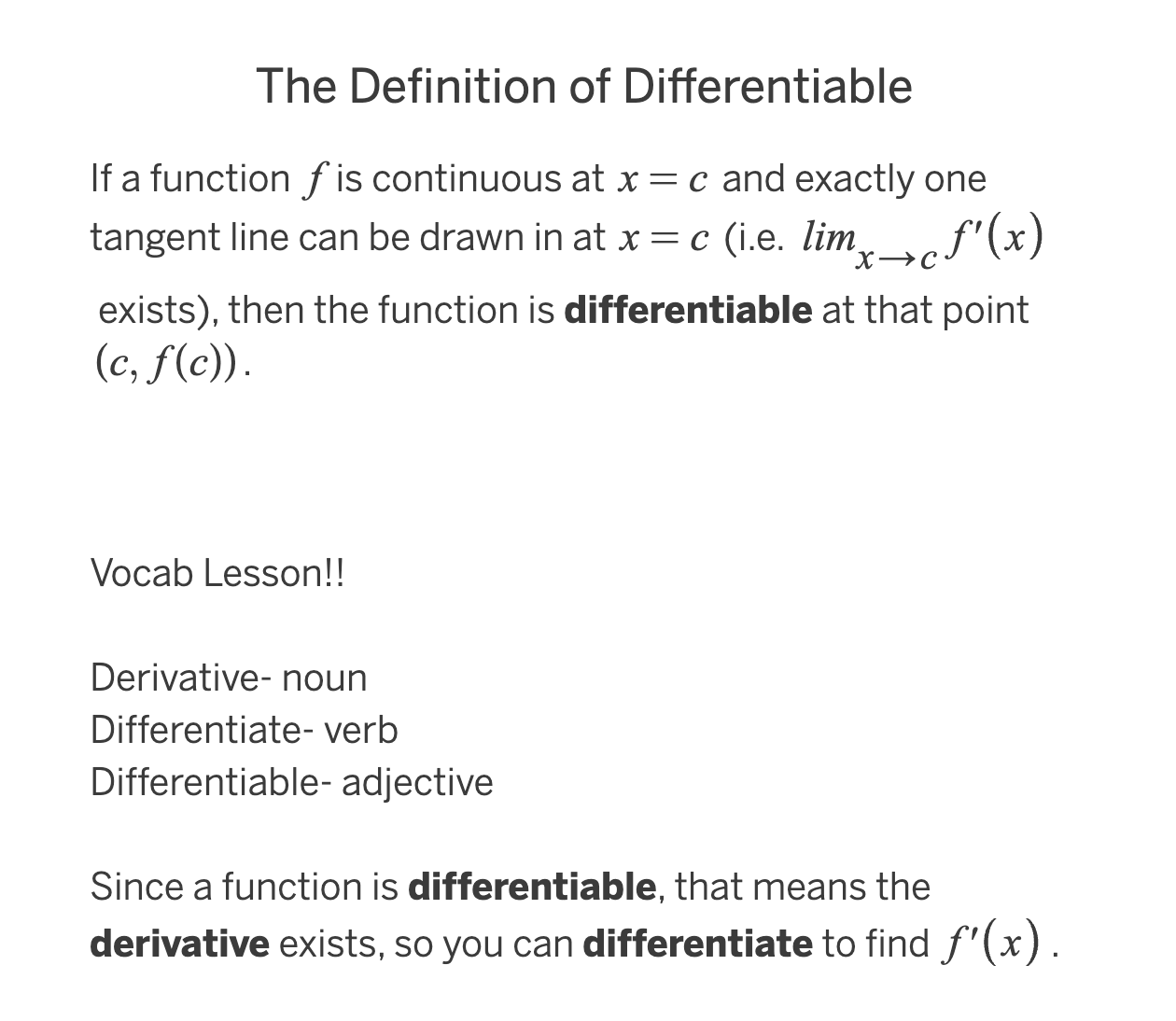
Definition: When a function is differentiable at all values of x in its domain, then it is said to be differential everywhere. A function that is twice differentiable is both differentiable everywhere and lim f”(x) as x approaches c exists everywhere
Rules for differentiability:
- f is continuous at x = c
- lim f’(x) exists at x -> c